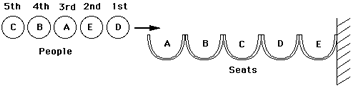|
Tools - Math 'Creating Measures' Awkward-ness Task, Example #5 (solution)
Square-ness, Example #1 (solution) || Steep-ness, Example #2 (solution)
Compact-ness, Example #3 (solution) || Crowded-ness, Example #4 (solution)
Awkward-ness, Example #5 (solution) || Sharp-ness, Example #6 (solution)
Malcolm Swan
Mathematics Education
University of Nottingham
Malcolm.Swan@nottingham.ac.uk
Jim Ridgway
School of Education
University of Durham
Jim.Ridgway@durham.ac.uk
This task gives you the chance to
- invent your own measure for the concept of "awkward-ness"
- use your measure to put situations in order of "awkward-ness"
- generalize your measure to work in different situations.
|
- So, D arrives first and sits in the second seat from the right hand end of the row.
- Then E arrives. D has to stand up while E squeezes into the last seat in the row.
- Then A arrives. She sits on the first seat of the row.
- Now B arrives and makes A stand, while he takes the second seat in.
- Finally C arrives and makes both A and B stand up while she takes her seat.
Warm-up
Try out this situation from different starting points using scraps of paper labeled A, B C, D and E until you can see what is happening.
What is the most awkward situation you can devise?
Draw it below:
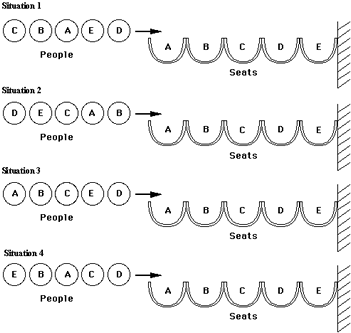
Here are four movie theater situations:
Comment:
The most awkard situation possible is shown below:
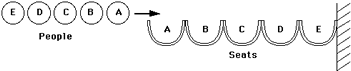
In this situation, A sits first, then
- A stands while B takes her place
- A and B stand while C takes his place
- A, B and C stand while D takes his place
- A, B, C and D stand while E takes her place.
- Place the four situations in order of "awkward-ness."
Which is the easiest situation for people?
Which is the most awkward?
Explain how you decided.
Solution:
The above measure is unsatisfactory because:
The easiest situation is situation (3), because this results in only one person having to stand on one occasion (person D has to stand while E squeezes by).
The most awkward situation is probably (4) because people have to stand on five occasions. (A has to stand while B sits down, then A, B, C and D all have to stand while E sits down.)
- Invent a way of measuring "awkward-ness." This should give a number to each situation. Explain carefully how your method works.
Solution for Questions 2 and 3:
A suitable measure of "awkward-ness" would be to count the number of times a person makes someone stand up to let them pass. This would give, for situations 1 to 4:
| |
Number of times person makes someone else stand |
| Situation |
A |
B |
C |
D |
E |
Total |
| 1 |
0 |
1 |
2 |
0 |
1 |
4 |
| 2 |
0 |
0 |
2 |
3 |
3 |
8 |
| 3 |
0 |
0 |
0 |
0 |
1 |
1 |
| 4 |
0 |
1 |
0 |
0 |
4 |
5 |
Using the totals, we have, from least to most awkward:
Situations 3, 1, 4 then 2.
- Show how you can use your measure to place the four situations in order of "awkward-ness." Show all your work.
- Adapt your measure so that the minimum value it can take is 0 (where no-one is made to stand up) and the maximum it can take is 1 (the most awkard situation possible).
Solution:
To make the measure range from 0 to 1, we could divide the totals above by the maximum possible "awkward-ness" score for five people = 10 (see Warm-up).
- Show how your measure in part 4 may be generalised for any number of people entering a row. ( That is when n people enter a row with n available seats).
Solution:
If there was just one person, the maximum "awkward-ness" = 0.
For 2 people, the maximum "awkward-ness" = 1.
For 3 people, the maximum "awkward-ness" = 3 (= 1 + 2).
For 4 people, the maximum "awkward-ness" = 6 (= 1 + 2 + 3).
For 5 people, the maximum "awkward-ness" = 10 (= 1 + 2 + 3 + 4).
...
For n people, the maximum "awkward-ness" = 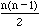 (= 1 + 2 + 3 + ... n). (= 1 + 2 + 3 + ... n).
Thus, if s = The number of occasions on which people have to stand;
we can define our measure of "awkward-ness" for a given situation to be:
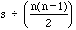
= 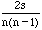
Square-ness, Example #1 (solution) || Steep-ness, Example #2 (solution)
Compact-ness, Example #3 (solution) || Crowded-ness, Example #4 (solution)
Awkward-ness, Example #5 (solution) || Sharp-ness, Example #6 (solution)

|