
|
Tools - Math 'Creating Measures' Steep-ness Task, Example #2 (solution)
Square-ness, Example #1 (solution) || Steep-ness, Example #2 (solution)
Compact-ness, Example #3 (solution) || Crowded-ness, Example #4 (solution)
Awkward-ness, Example #5 (solution) || Sharp-ness, Example #6 (solution)
Malcolm Swan
Mathematics Education
University of Nottingham
Malcolm.Swan@nottingham.ac.uk
Jim Ridgway
School of Education
University of Durham
Jim.Ridgway@durham.ac.uk
This problem gives you the chance to:
- criticise a given measure for the concept of "steep-ness"
- invent your own ways of measuring this concept
- examine the advantages and disadvantages of different methods.
|
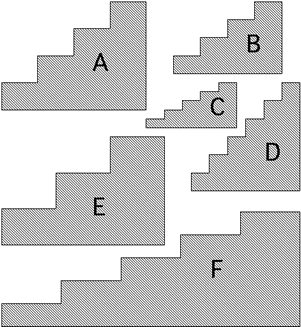
Warm-up
Without measuring anything, put the above staircases in order of "steep-ness."
Comment:
This first question is simply intended to orientate the students to the task. It may be used as a class introduction.
- Someone has suggested that a good measure of "steep-ness" is to calculate the difference:
Height of step - length of step
for each staircase. Use this definition to put the staircases in order of "steep-ness."
Show all your work.
Solution:
Using the measure 'height of each step - length of each step', the 'steep-ness' of each staircase is given in the table below (using centimeters as the unit).
| Staircase |
A |
B |
C |
D |
E |
F |
| Height (cm) |
1.5 |
1 |
0.5 |
1 |
2 |
1.25 |
| Length (cm) |
2 |
1.5 |
1 |
1 |
3 |
3.33 |
| Height-length (cm) |
-0.5 |
-0.5 |
-0.5 |
0 |
-1 |
-2.08 |
Using this measure, the staircases in order from most to least steep are:
D, A and B and C (tie), E, F.
- Using your results, give reasons why Height of step - length of step is not a suitable measure for "steep-ness."
Solution:
The above measure is unsatisfactory because:
- It gives no real indication of the steepness. Using this measure, A and C are labeled as equally steep, which does not fit with intuition.
- It is dependent on the units used. If we use inches instead of centimetres we get a different "steep-ness" measure.
- It is usually negative, which is inelegant and awkward to use.
- Invent a better way of measuring "steep-ness." Describe your method carefully below:
Solution:
There are many other ways of measuring "steep-ness." Students might, for example, propose using:
- The angle of inclination;
- The ratio of 'step height'/'step length' (technically: riser/run);
- The ratio of 'height of whole staircase'/ 'length of whole staircase';
These are equally sensible, and equivalent, except is may be sometimes unclear what we measure as the 'length' of the staircase.
- Place the staircases in order of "steep-ness" using your method. Show all your work.
Solution:
Whichever measure we now use (a), (b) or (c), we obtain the same order for the staircases.
| Staircase |
A |
B |
C |
D |
E |
F |
| Height (cm) |
1.5 |
1 |
0.5 |
1 |
2 |
1.25 |
| Length (cm) |
2 |
1.5 |
1 |
1 |
3 |
3.33 |
Height ÷ length
(2 d.p.) |
0.75
(3/4) |
0.67
(2/3) |
0.5
(1/2) |
1
(1/1) |
0.67
(2/3) |
0.38
(3/8) |
| Angel of inclination (nearest degree) |
37o |
34o |
27o |
45o |
34o |
21o |
This gives the order of steep-ness (from most to least steep) as:
D, A, B and E (tie), C and F.
- Do you think your measure is a good way of measuring "steep-ness?" Explain your reasoning carefully.
Solution:
Here we would like students to review their results critically and decide whether the results from their measurements accord with their intuitions.
- Describe a different way of measuring "steep-ness."
Compare the two methods you invented. Which is best? Why?
Solution:
This question provides an opportunity for students to look for an alternative measure.
Square-ness, Example #1 (solution) || Steep-ness, Example #2 (solution)
Compact-ness, Example #3 (solution) || Crowded-ness, Example #4 (solution)
Awkward-ness, Example #5 (solution) || Sharp-ness, Example #6 (solution)

|

